Three views of Fire Series [0352], computational origami by Erik Demaine and Martin Demaine via erikdemaine.org
–
Since the word computation harks back to the 1400s you might reason that the modern efflorescence of “computational” arts and sciences was based in mathematical calculations. Half right. Other half is the extra-human capacity of computers which now take those calculations to an nth degree.
Mathematically possible but previously unattainable numbers are now being plumbed. Computational has come to mean math-based at a new and as yet unfamiliar scope: titanic. Computational origami, computational botany, computational medicine. Name your subject and slap Computational on the front. Now you’re an explorer who asks where you can travel in your field powered by rocket math. Boundaries in many fields will change. Terra incognita: reshaped.
What’s the nth degree? For our purposes it’s a number that requires a computer’s power to investigate. Say you want to add 1 + 1 + 1 + 1 + 1 + 1 + 1… If you hypothesize doing this six trillion times you don’t need a computer to tell you the answer is six trillion.
How about the old tale of the rice grains and the chessboard? The king is owed a lot of gold by an enemy but he offers to let the debtor off the hook thus: he drops a grain of rice on the 1st square of a chessboard, then two on the 2nd square. All the debtor must do is to continue the pattern until the last square is filled.
Will the debtor be fool enough to agree? Here is an illustration showing the one grain as it doubles around the board. The grains will ultimately total to 18,446,744,073,709,551,615. Eighteen quintillion, four hundred forty six quadrillion, seven hundred forty four trillion, seventy three billion, seven hundred nine million, five hundred fifty thousand, six hundred fifteen — reached by an exponential growth that starts at 2 grains and piles up to 263.
Grains and Chessboard Problem via Wikipedia [More about the red line at the bottom of this page.]
Think of having to pay for the oxcarts alone. Yet this is a number first recorded by the Persian poet Ferdowsi before the year 1011. Recorded. Already humanity had developed sufficient mathematical tools to solve the problem. (How the poet got it to scan inside his poem I don’t know.)
Consider this when you approach the new computational arts and sciences. They’re truly pushing into territories where humans have never been able to nose around before.
_______________________________________
Computational Origami is for modeling the ways in which various materials, including paper, can be folded. [see more below]
Erik Demaine and his father Martin Demaine, both faculty at MIT, create Curved Crease sculptures which are shown by galleries and museums.
Pushing Curves to the Limit, #5 by Erik Demaine and Martin Demaine via erikdemaine.org
Earthtone Series, [0262] by Erik Demaine and Martin Demaine via erikdemaine.org
Simons Series [0238] by Erik Demaine and Martin Demaine via erikdemaine.org
From Kentucky Series by Erik Demaine and Martin Demaine via erikdemaine.org
Anni Albers, pioneering textile designer and wife of Bauhaus luminary Joseph Albers:
“Being creative is not so much the desire to do something as the listening to that which wants to be done: the dictation of the materials.” Anni Albers quoted on improvisedlife
Pushing Curves to the Limit, #4, by Erik Demaine and Martin Demaine via erikdemaine.org
Each piece is a combination of between two and four units, where each unit is hand-folded from one circle of paper with a circular hole and hand-scored concentric circular creases. The pieces are combined by joining a few key points together; the rest of the shape is formed from the natural equilibrium of the paper. Erik Demaine, Pushing Curves to the Limit
The dictation of the materials and the natural equilibrium of the paper. Yes. The origamist’s choice of paper influences the form and feel of every finished piece. Does it slump gracefully? Hold a pose with military starch? Is it translucent, thick and grainy, Kraft-paper brown, flecked with mylar sparkle?
Imagine how future computational origamists will use math and eye to incorporate patterned paper into an even higher order design.
Hexagon wallpaper design, Cole & Son via designerwallpapers.co.uk
_______________________________________
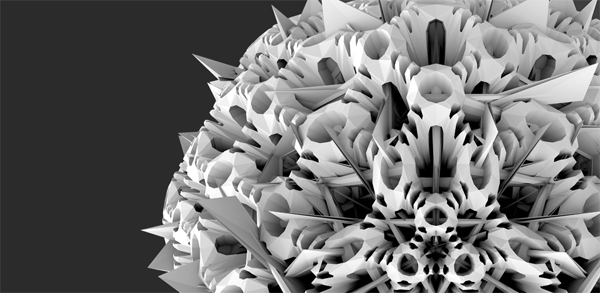
Michael Hansmeyer’s computational architecture inhabits a whole different realm. Compare the wallpaper hexagrams just above with his Stacked Hexahedra below.
Stacked Hexahedra by Michael Hansmeyer via michael-hansmeyer.com
This project begins with the most primitive forms, the platonic solids, and repeatedly employs a single operation – the division of a form’s faces into smaller faces – until forms of an astounding complexity are produced. Michael Hansmeyer
Dodecahedron by Michael Hansmeyer via michael-hansmeyer.com
For the first time, complexity is not an impediment to design and fabrication. Rather, it is an opportunity that is waiting to be explored. For years, it was information technology that constrained architects. Arguably, this relationship has reversed: it is now architects who are constraining the possibilities of information technology. Michael Hansmeyer
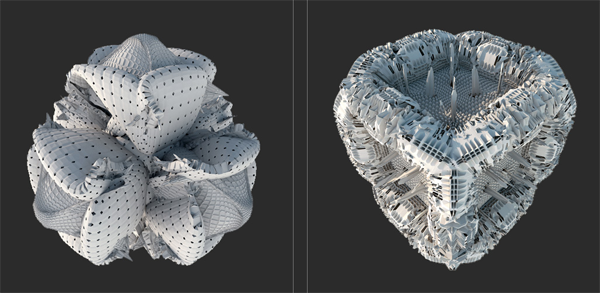
Tetrahedron and Hexahedron by Michael Hansmeyer via michael-hansmeyer.com
These last two by Hansmeyer are obviously from other series.
Pavilion 4 (Catmull-Clark subdivision), by Michael Hansmeyer via michael-hansmeyer.com
1:10 Prototype Gilded by Michael Hansmeyer via michael-hansmeyer.com
Hansmeyer’s work brings neo-Gothic and neo-Gaudi structures closer to our built reality.
look further
Red line on the chessboard diagram
- • “Second half of the chessboard.” In technology strategy, the second half of the chessboard is a phrase, coined by Ray Kurzweil, in reference to the point where an exponentially growing factor begins to have a significant economic impact on an organization’s overall business strategy.
- • While the number of grains on the first half of the chessboard is large, the amount on the second half is vastly (232 > 4 billion times) larger.
Ferdowsi
- • Hakīm Abul-Qāsim Ferdowsī Tūsī wrote the Shahnameh, Book of Kings, an epic poem and masterpiece of Persian literature.
Relevant rice
- • MASS MoCA Installation Comprises more than 800 Million Grains of Rice, via iBerkshires.com
-
875,000,000 grains… will be used to dramatize the enormity of the population of the Americas and the miniscule place of one person in it. (All the rice will be recycled, and will re-enter the food chain upon completion of the performance.) Far from a static display, this landscape will constantly change as performers from the UK artists collective pile, measure, sort, and re-pile the rice to represent different comparative statistics, population changes, and demographic anomalies.
Computational origami
- • (continued from description above)The principles of geometry were first applied to origami around the mid-twentieth century, when Japanese physicists and mathematicians began to formulate axioms… that explain how folding creates three dimensional objects from a flat material. Humaiki Huzita, an Italian-Japanese mathematician, developed a sequence of six increasingly complex origami axioms that describe, at the most basic level, how any two points on a flat surface can be connected in a single line fold, and at the most complex level, the ways that four points on a flat surface can be related.Computational origami has been used to create complex paper objects, such as insects, that were once thought to be beyond the medium’s capacities. In addition… how to most effectively fold a roadmap, an airbag, and computer processors. The latter purpose was one of the original driving forces behind the development of computational origami: researchers believe that by folding processors most efficiently, they can fit the maximum amount of information into the smallest possible area. Margaret Rouse, via WhatIs.
- –
- • Robert J. Lang Origami Generous and broad information on computational origami.
- • Robert J. Lang Lectures and Workshops
Erik Demaine
- • Classes, videos and teaching here
- • Upcoming events
Anni Albers